Page 167 - Maths Class 06

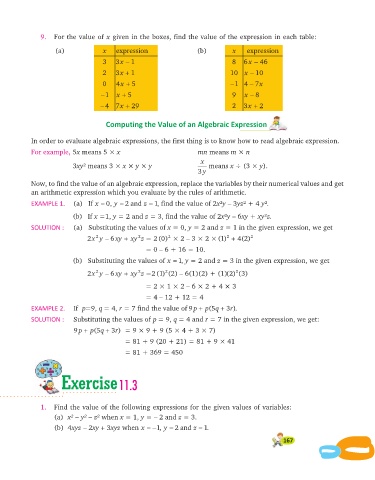
9. For the value of x given in the boxes, find the value of the expression in each table:

(a) x expression (b) x expression

3 3x – 1 8 6x - 46
2 3x + 1 10 x - 10
0 4x + 5 -1 4 - 7x

-1 x + 5 9 x - 8
-4 7x + 29 2 3x + 2

Computing the Value of an Algebraic Expression

In order to evaluate algebraic expressions, the first thing is to know how to read algebraic expression.
For example, 5x means 5 × x mn means m × n
x
3xy² means 3 × x × y × y means x ÷ (3 × y).
3 y

Now, to find the value of an algebraic expression, replace the variables by their numerical values and get
an arithmetic expression which you evaluate by the rules of arithmetic.
EXAMPLE 1. (a) If x = 0, y = 2 and z = 1, find the value of 2x²y – 3yz² + 4 y².

(b) If x =1, y = 2 and z = 3, find the value of 2x²y – 6xy + xy²z.
SOLUTION : (a) Substituting the values of x = 0, y = 2 and z = 1 in the given expression, we get

2
2
2
2
2x y – 6xy + xy z = 2 ( )0 × 2 – 3 × 2 × ( )1 + 4 2( ) 2
= 0 – 6 + 16 = 10.
(b) Substituting the values of x = 1, y = 2 and z = 3 in the given expression, we get
2
2
2
2
2x y – 6xy + xy z =2 ( )1 (2) – 6(1)(2) + (1)( )2 (3)
= 2 × 1 × 2 – 6 × 2 + 4 × 3
= 4 – 12 + 12 = 4
EXAMPLE 2. If p=9, q = 4, r = 7 find the value of 9p + p( 5q + 3r).
SOLUTION : Substituting the values of p = 9, q = 4 and r = 7 in the given expression, we get:

9p + p( 5q + 3r) = 9 × 9 + 9 (5 × 4 + 3 × 7)
= 81 + 9 (20 + 21) = 81 + 9 × 41
= 81 + 369 = 450

Exercise11.3

1. Find the value of the follow ing expressions for the given values of variables:
(a) x² – y² – z² when x = 1, y = - 2 and z = 3.

(b) 4xyz - 2xy + 3xyz when x = -1, y = 2 and z = 1.
167